How to Calculate the Magnitude and Direction of a Vector

Video Lesson: How to Calculate the Magnitude and Direction of a Vector

How to Calculate the Magnitude of a Vector

The magnitude of a vector is a measure of its size or length. To calculate the magnitude, use the formula |v| = √(x2 + y2) where x and y are the horizontal and vertical components of the vector respectively.

the absolute value of v equals the square root of x squared plus y squared

Formula for calculating the magnitude of a vector
how to find the magnitude of a vector

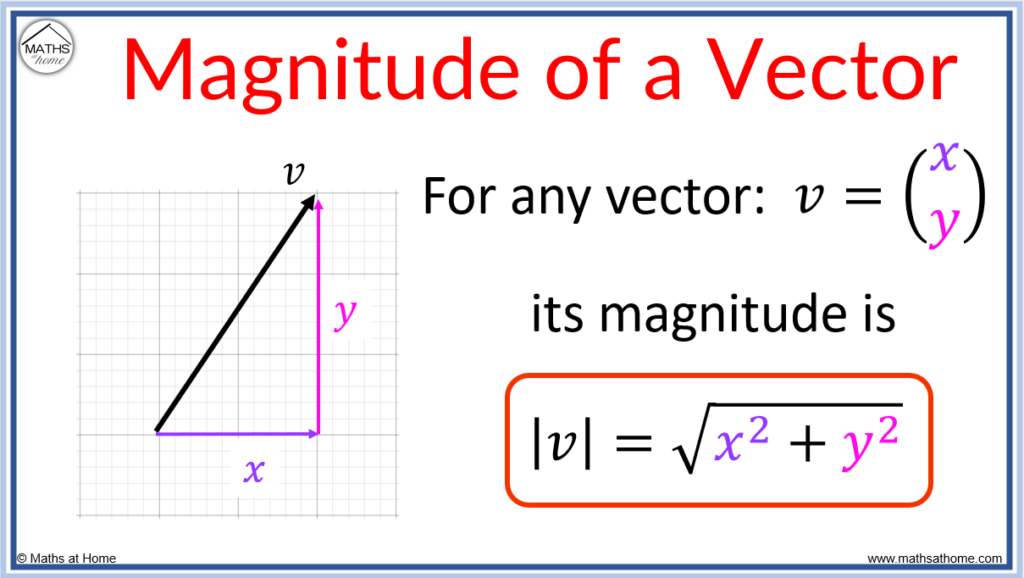
The magnitude of a vector is equal to its length and is a scalar quantity. The magnitude does not describe the direction of the vector.

The magnitude of a vector cannot be negative. All vector magnitudes are positive except for the zero vector which has a magnitude of zero.

How to Calculate the Magnitude of a 2D Vector

The magnitude of a 2D vector is calculated with |v| = √(x2 + y2), where x and y are the components of the vector.

For example, the magnitude of the vector v=(1, 4) is |v| = √(12 + 42) = √17.

The square root of 17 is approximately 4.12 and so, the length of the vector (1, 4) is approximately 4.12.

It is common to leave the magnitude of a vector written as a surd inside the square root in exact form.

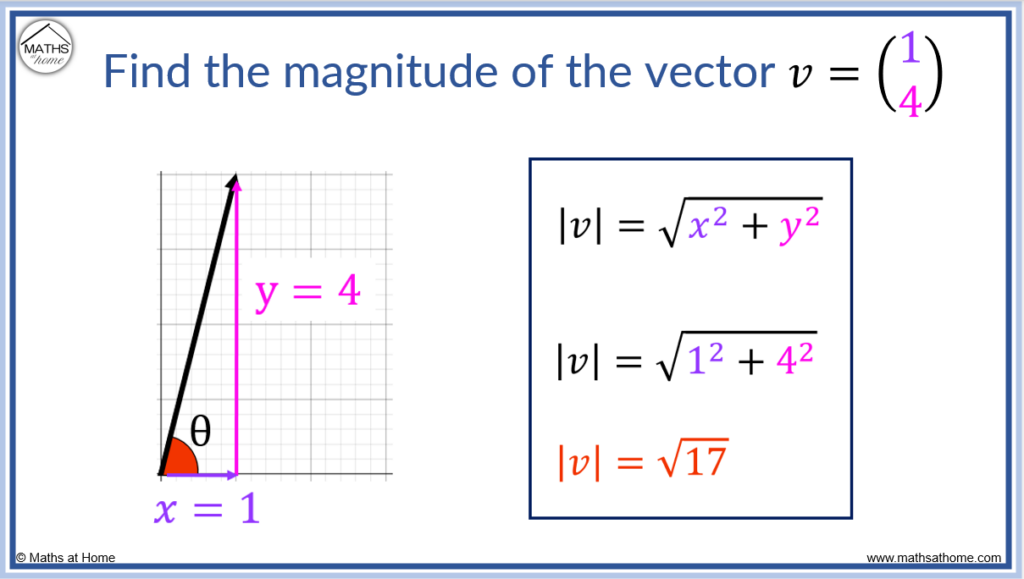
example of how to find the magnitude of a vector

Here are some examples of how to calculate the magnitude of a 2 dimensional vector:

VectorMagnitude CalculationMagnitude
(5, 1)√(52+12)√26
(3, 4)√(32+42)5
(0, 0)√(02+02)0
(-1, -2)√((-1)2+(-2)2)√5
(2, 2)√(22+22)√8

How to Calculate the Magnitude of a 3D Vector

To calculate the magnitude of a 3D vector v=(x, y, z), use the formula |v| = √(x2+y2+z2).

For example, the magnitude of the 3D vector (2, -1, 3) is √(22+(-1)2+32) which equals √14.

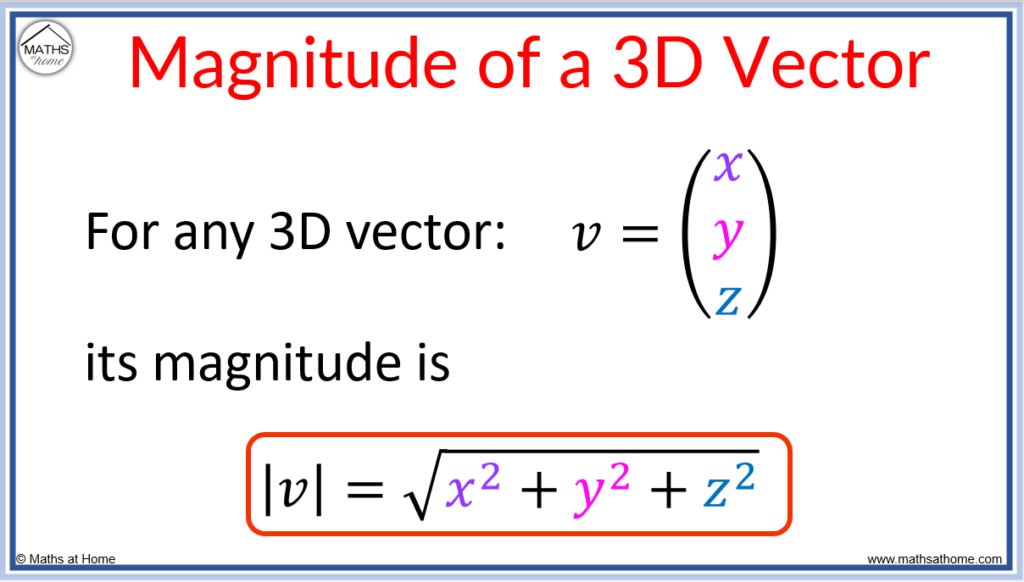
how to calculate the magnitude of a 3d vector
example of finding the magnitude of a 3d vector

The magnitude of a 3 dimensional vector is calculated using the Pythagorean theorem in the same way as a 2D vector but it involves one extra z component.

  • x is the x-component of the vector
  • y is the y-component of the vector
  • z is the z-component of the vector

the absolute value of v equals the square root of x squared plus y squared plus z squared

Formula for the magnitude of a 3 dimensional vector

Here are some examples of calculating the magnitude of a 3D vector:

VectorMagnitude CalculationMagnitude
(1, 3, 5)√(12+32+52)√35
(3, 0, 1)√(32+02+12)√10
(-1, -2, -3)√((-1)2+(-2)2+(-3)2)√14
(0,0,0)√(02+02+02)0
(10, 10, 10)√(102+102+102)√300

How to Calculate the Direction of a Vector

To calculate the direction of the vector v = (x, y), use the formula θ = arctan(y/x).

For vectors in quadrant 1, the direction is θ, in quadrant 2 the direction is 180° – θ, in quadrant 3 the direction is 180° + θ and in quadrant 4 the direction is 360° – θ.

how to find the direction of a vector

The direction of a vector is the angle measured counter-clockwise from the positive x-axis direction. The angle θ is the angle made between the x and y components of the vector.

Therefore the direction of the vector depends on the quadrant that the vector is in.

definition of the direction of a vector

How to Calculate the Direction of a 2D Vector

theta equals the inverse tangent of open paren y over x close paren

Formula for the direction of a 2 dimension vector

For example, calculate the direction of the vector v = (1, 4).

  • The x component is 1
  • The y component is 4

Therefore theta equals the inverse tangent of four oneths and so, theta equals 76 degrees.

Since the vector is in the first quadrant, theta equals 76 degrees is the direction of the vector.

example of how to find the direction of a vector

Calculate the direction of the vector (-2, -3).

  • The x component of the vector is -2
  • The y component of the vector is -3

The vector is in quadrant two and therefore, the direction is equal to 180 degrees plus theta.

theta equals the inverse tangent of open paren y over x close paren and so, theta equals the inverse tangent of open paren negative 3 over negative 2 close paren.

Evaluating this, theta equals 56.3 degrees and therefore the direction is 180 degrees plus 56.3 degrees equals 236.3 degrees.

example of how to calculate the direction of a 2d vector

Vector Magnitude and Direction Calculator

The following calculator will calculate the magnitude and direction of a vector.

For any vector v equals the 2 by 1 column matrix x y, enter the x component and y component in the calculator below to obtain the magnitiude and direction.