The Complete Guide to the Trigonometry Double Angle Formulas

Video Lesson: What are the Double Angle Formulas?

Video Lesson: How to Use the Double Angle Formulas

What are the Double Angle Formulae?

The double angle formulae are:

  • sin(2θ)=2sin(θ)cos(θ)
  • cos(2θ)=cos2θ-sin2θ
  • tan(2θ)=2tanθ/(1-tan2θ)

The double angle formulae are used to simplify and rewrite expressions, allowing more complex equations to be solved. They are also used to find exact trigonometric values for multiples of a known angle.

Double Angle Formulae List

Here is a list of the double angle formulae.

double angle formula list
  • The double angle formula for sine is sine 2 theta equals 2 sine theta cosine theta.
  • The double angle formula for cosine is 1 lines Line 1: cosine 2 theta equals cosine squared theta minus sine squared theta. This can also be written as 1 lines Line 1: cosine 2 theta equals 1 minus 2 sine squared theta or 1 lines Line 1: cosine 2 theta equals 2 cosine squared theta minus 1.
  • The double angle formula for tangent is 1 lines Line 1: tangent 2 theta equals the fraction with numerator 2 tangent theta and denominator 1 minus tangent squared theta.

Double Angle Formula Derivation

To derive the double angle formulas, start with the compound angle formulas, set both angles to the same value and simplify. The best way to remember the double angle formulas is to derive them from the compound angle formulas.

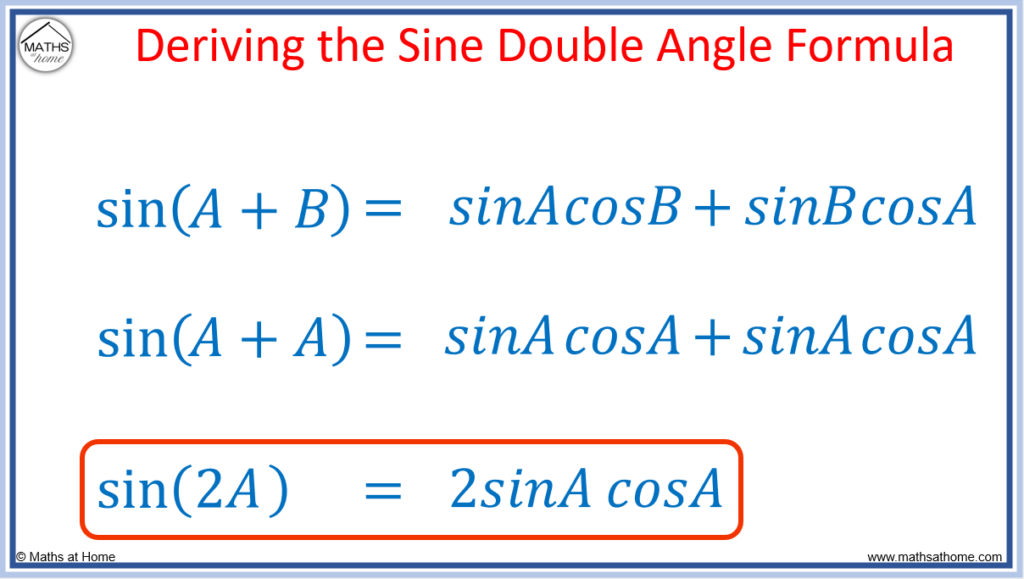
Double Angle Formula for Sine

To derive the sine double angle formula, sine 2 A equals 2 sine A cosine A

  • Start with the compound angle formula the sine of open paren A plus B close paren equals sine A cosine B plus sine B cosine A
  • Substitute B=A to obtain the sine of open paren A plus A close paren equals sine A cosine A plus sine A cosine A
  • This simplifies to sine 2 A equals 2 sine A cosine A
deriving the sine double angle formula

Double Angle Formula for Cosine

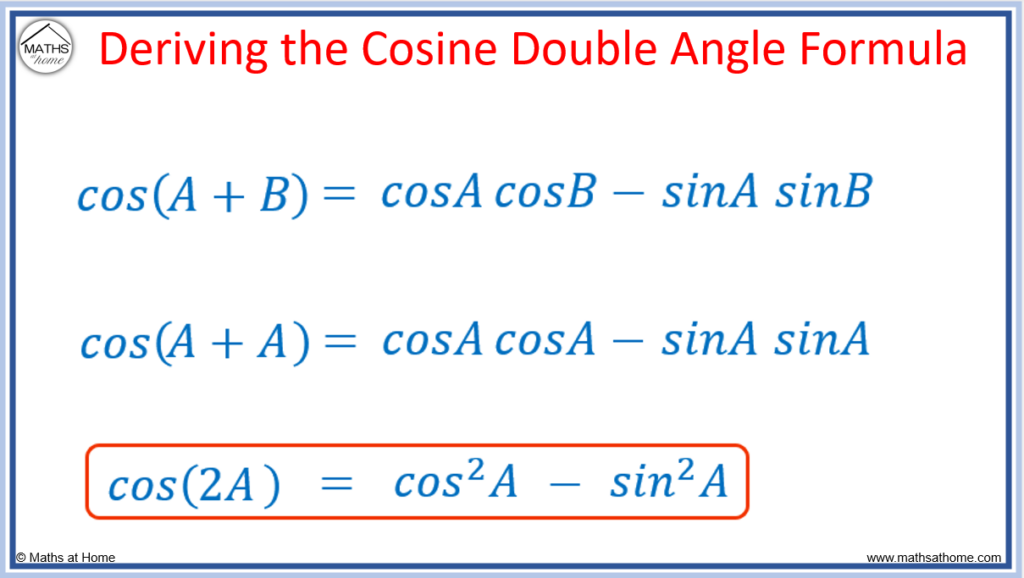
To derive the cosine double angle formula, cosine 2 A equals cosine squared A minus sine squared A

  • Start with the cosine compound angle formula, the cosine of open paren A plus B close paren equals cosine A cosine B minus sine A sine B
  • Substitute B=A to obtain the cosine of open paren A plus A close paren equals cosine A cosine A minus sine A sine A
  • Simplify to obtain cosine 2 A equals cosine squared A minus sine squared A
deriving the cosine double angle formula

The cosine double angle formula can be rewritten in two different forms using the Pythagorean identity sine squared theta plus cosine squared theta equals 1.

sine squared theta plus cosine squared theta equals 1 can be rearranged as either:

  • sine squared theta equals 1 minus cosine squared theta
  • cosine squared theta equals 1 minus sine squared theta

To rearrange the double angle formula for cosine, substitute sine squared theta equals 1 minus cosine squared theta into

This becomes cosine 2 theta equals cosine squared theta minus open paren 1 minus cosine squared theta close paren.

Simplifying, this becomes cosine 2 theta equals 2 cosine squared theta minus 1.

Alternatively, the double angle formula for cosine can be rearranged by substituting cosine squared theta equals 1 minus sine squared theta into cosine 2 theta equals cosine squared theta minus sine squared theta.

This becomes cosine 2 theta equals open paren 1 minus sine squared theta close paren minus sine squared theta.

Simplifying, this becomes cosine 2 theta equals 1 minus 2 sine squared theta.

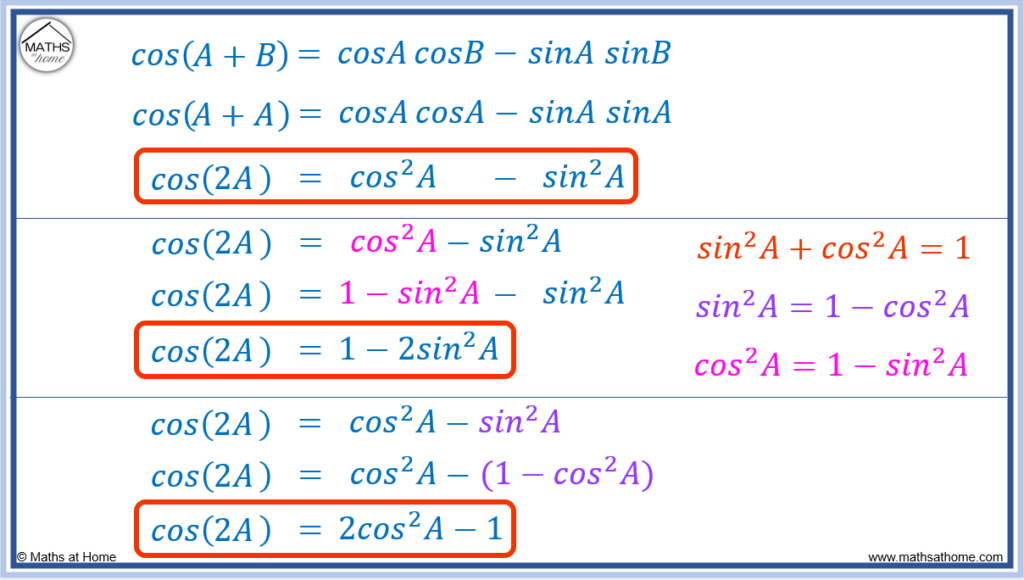
double angle formulae for cosine

The double angle formula for cosine is written in the following three ways:

  • cosine 2 theta equals cosine squared theta minus sine squared theta
  • cosine 2 theta equals 1 minus 2 sine squared theta
  • cosine 2 theta equals 2 cosine squared theta minus 1

Double Angle Formula for Tangent

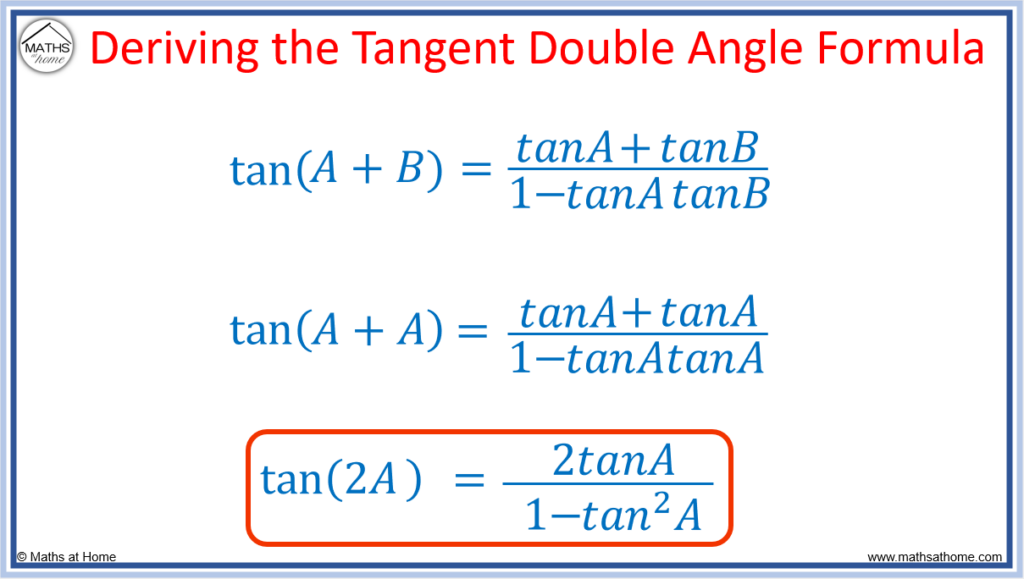
To derive the tangent double angle formula, tangent 2 A equals the fraction with numerator 2 tangent A and denominator 1 minus tangent squared A

  • Start with the tangent compound angle formula, the tangent of open paren A plus B close paren equals the fraction with numerator tangent A plus tangent B and denominator 1 minus tangent A tangent B
  • Substitute B=A to obtain the tangent of open paren A plus A close paren equals the fraction with numerator tangent A plus tangent A and denominator 1 minus tangent A tangent A
  • Simplify to obtain tangent 2 A equals the fraction with numerator 2 tangent A and denominator 1 minus tangent squared A
deriving the tangent double angle formula

Rewriting Expressions Using the Double Angle Formulae

To simplify expressions using the double angle formulae, substitute the double angle formulae for their single-angle equivalents. The tanx=sinx/cosx and the Pythagorean trigonometric identity of sin2x+cos2x=1 may also be needed.

Example 1

Show that the fraction with numerator sine 2 theta and denominator 1 plus cosine 2 theta equals tangent theta.

We start by substituting the two double angle formulae for sine and cosine.

  • sine 2 theta equals 2 sine theta cosine theta
  • cosine 2 theta equals cosine squared theta minus sine squared theta or cosine 2 theta equals 2 cosine squared theta minus 1 or cosine 2 theta equals 1 minus 2 sine squared theta

We will choose to substitute the cosine 2 theta equals 1 minus 2 sine squared theta equation because subtracting the1 will cancel out the 1 already on the denominator.

the fraction with numerator sine 2 theta and denominator 1 plus cosine 2 theta becomes the fraction with numerator 2 sine theta cosine theta and denominator 1 minus open paren 1 minus 2 sine squared theta close paren.

This simplifies to the fraction with numerator 2 sine theta cosine theta and denominator 2 sine squared theta. We can now divide the numerator and denominator both by 2 sine theta to obtain cosine theta over sine theta which equals tangent theta.

Example 2

Show that the fraction with numerator cosine 2 theta and denominator sine theta plus cosine theta equals cosine theta minus sine theta.

We start with the left hand side of the equation, substituting the double angle formula of cosine 2 theta equals cosine squared theta minus sine squared theta.

The equation becomes the fraction with numerator cosine squared theta minus sine squared theta and denominator sine theta plus cosine theta equals cosine theta minus sine theta.

Looking at the fraction, there is nothing we can divide each term by. Instead we can multiply both sides of the fraction by the denominator of sine theta plus cosine theta.

the fraction with numerator cosine squared theta minus sine squared theta and denominator sine theta plus cosine theta equals cosine theta minus sine theta becomes cosine squared theta minus sine squared theta equals open paren sine theta plus cosine theta close paren times open paren cosine theta minus sine theta close paren.

Expanding the brackets on the right hand side of the equation, cosine squared theta minus sine squared theta equals cosine squared theta plus sine theta cosine theta minus sine theta cosine theta minus sine squared theta.

This simplifies so that the left hand side equals the right hand side: cosine squared theta minus sine squared theta equals cosine squared theta minus sine squared theta.

Example 3

Show that 2 cosec of 2 theta equals sine theta over cosine theta plus cosine theta over sine theta.

We avoid the temptation to replace sine theta over cosine theta equals tangent theta as tangent theta does not feature on the left hand side of the equation. Instead, we will use the double angle formula.

2 cosec of 2 theta equals 2 over sine 2 theta

We will substitute sine 2 theta equals 2 sine theta cosine theta so that 2 over sine 2 theta equals 2 over 2 sine theta cosine theta.

We can simplify this fraction by dividing the numerator and denominator by 2 to obtain 1 over sine theta cosine theta.

So far, the equation becomes 1 over sine theta cosine theta equals sine theta over cosine theta plus cosine theta over sine theta.

We will add the fractions on the right hand side of the equation so that they become one fraction.

1 over sine theta cosine theta equals sine squared theta over sine theta cosine theta plus cosine squared theta over sine theta cosine theta equals the fraction with numerator sine squared theta plus cosine squared theta and denominator sine theta cosine theta

Using the Pythagorean trigonometric identity, sine squared theta plus cosine squared theta equals 1, we obtain 1 over sine theta cosine theta equals 1 over sine theta cosine theta.

Finding Exact Values Using the Double Angle Formulae

Example 1

If tangent theta equals three fourths find the exact value of tangent 2 theta.

Since tangent 2 theta equals the fraction with numerator 2 tangent theta and denominator 1 minus tangent squared theta, simply substitute tangent theta equals three fourths into this equation.

tangent 2 theta equals the fraction with numerator 2 times three fourths and denominator 1 minus three fourths squared

Evaluating this, we obtain tangent 2 theta equals 24 over 7.

The answer is left as a fraction to leave it in exact form.

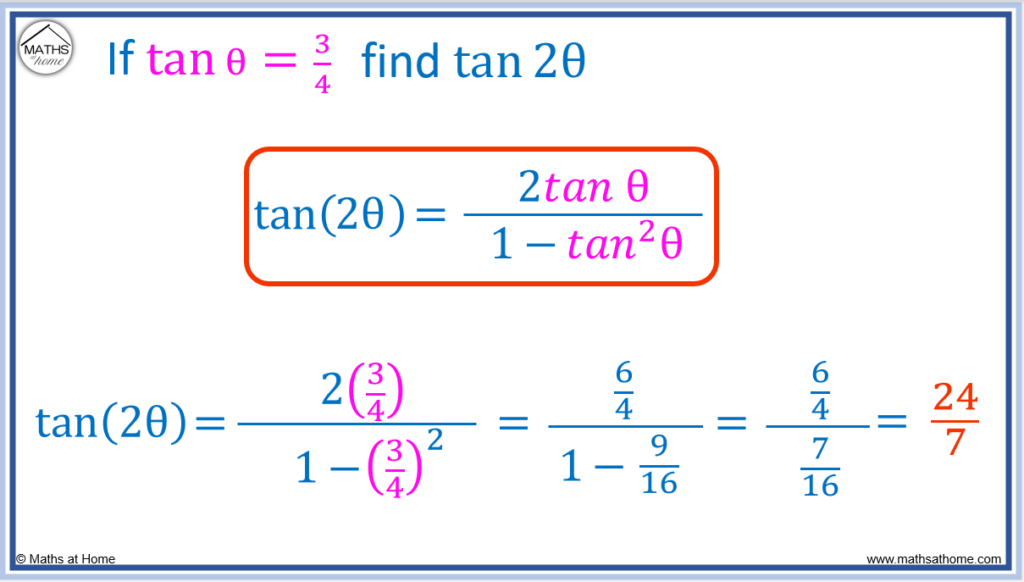
using the tan double angle formula to find an exact value of tan2a

Example 2

When calculating exact trigonometric values, it is important to know which quadrant the angle given is in.

Angles between 0 is less than theta is less than pi over 2 are in quadrant 1
Angles between pi over 2 is less than theta is less than pi are in quadrant 2
Angles between pi is less than theta is less than 3 pi over 2 are in quadrant 3
Angles between 3 pi over 2 is less than theta is less than 2 pi are in quadrant 4

angle quadrants

If tangent theta equals three fourths, find the exact value of sine 2 theta, where 0 is less than theta is less than pi over 2.

Since the angle is between 0 is less than theta is less than pi over 2, we draw a triangle in the first quadrant.

Since tangent theta equals o p p o s i t e over a. d j a. c e n t and tangent theta equals three fourths, then the opposite side must be 3 and the adjacent side must be 4.

We label those sides on the triangle.

We then use Pythagoras’ theorem to find the hypotenuse.

H equals the square root of 4 squared plus 3 squared equals 5

Now that we know all three sides of the triangle, we can find sine theta and cosine theta.

sine theta equals the fraction with numerator o p p o s i t e and denominator h of y p o t e n u s e equals three fifths and cosine theta equals the fraction with numerator a. d j a. c e n t and denominator h of y p o t e n u s e equals four fifths

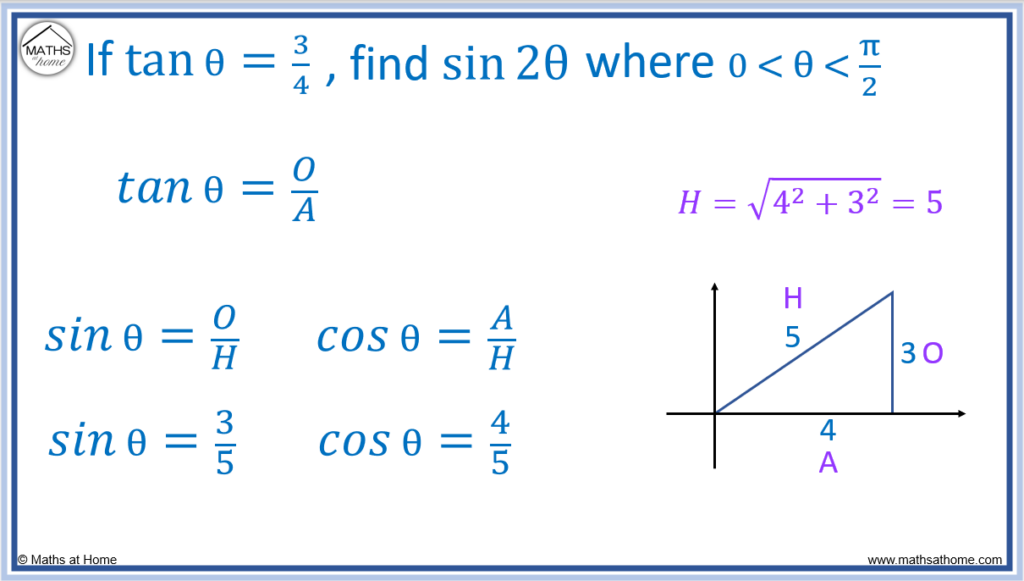
finding sin and cos given tan

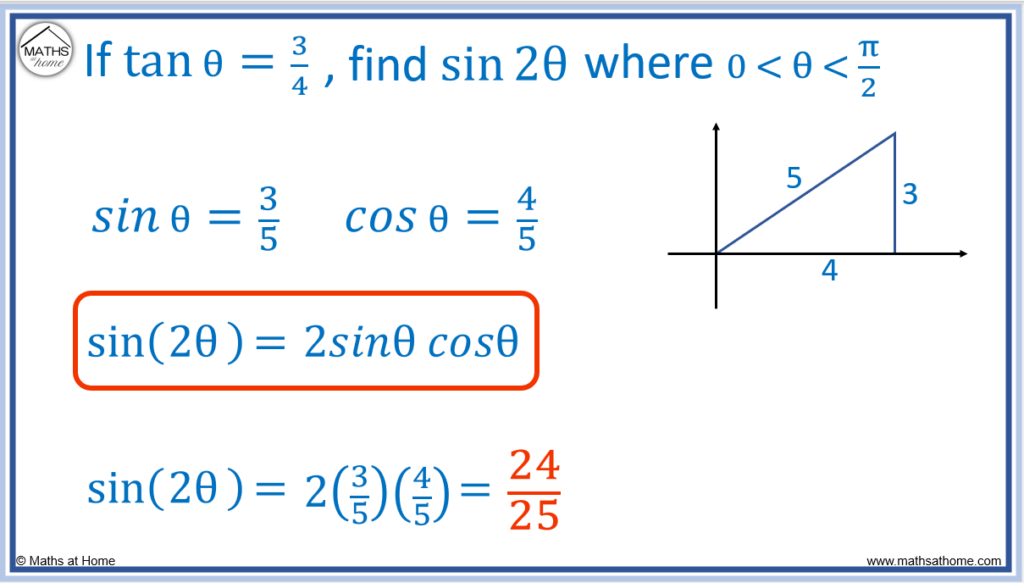
Now sine 2 theta can be found using the double angle formula sine 2 theta equals 2 sine theta cosine theta.

Since sine theta equals three fifths and cosine theta equals four fifths, the double angle formula becomes sine 2 theta equals 2 times three fifths times four fifths.

Evaluating this, sine 2 theta equals 24 over 25.

double angle formula to find exact values

Example 3

If cosine theta equals 12 over 13, find secant 2 theta where 3 pi over 2 is less than theta is less than 2 pi.

With 3 pi over 2 is less than theta is less than 2 pi, the angle is in quadrant 4. The triangle is drawn below.

Since cosine theta equals the fraction with numerator a. d j a. c e n t and denominator h of y p o t e n u s e and cosine theta equals 12 over 13, the adjacent = 12 and the hypotenuse = 13.

Using Pythagoras theorem, o p p o s i t e equals the square root of 13 squared minus 12 squared equals 5. Since the opposite side is below the x-axis, the opposite side length is -5.

Now that all sides of the triangle are known, sine theta equals the fraction with numerator o p p o s i t e and denominator h of y p o t e n u s e equals negative 5 over 13.

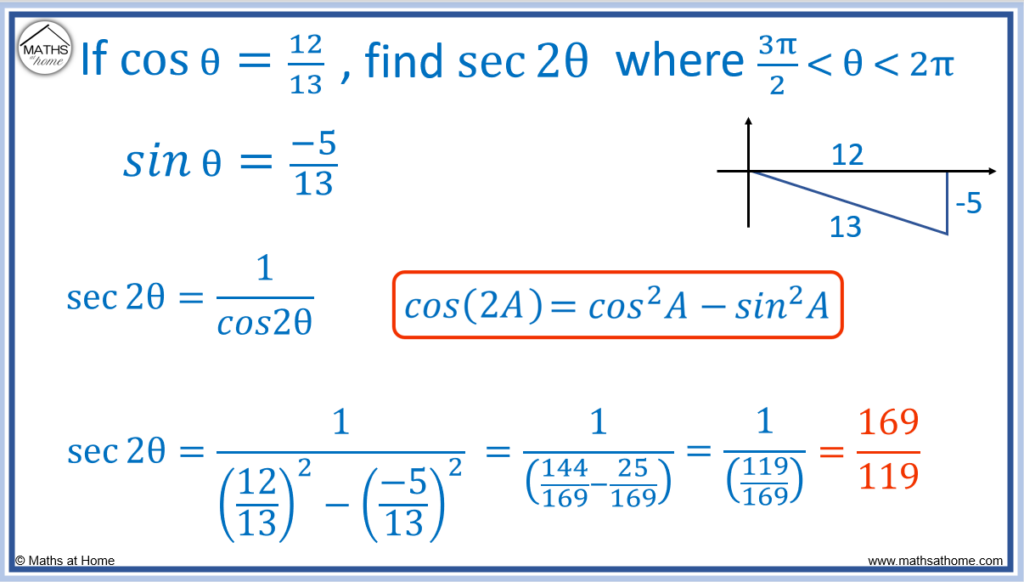
double angle formula to find exact trig values

secant 2 theta equals 1 over cosine 2 theta.

Using the double angle formula, cosine 2 theta equals cosine squared theta minus sine squared theta, 1 over secant 2 theta equals 1 over cosine 2 theta equals the fraction with numerator 1 and denominator cosine squared theta minus sine squared theta.

Substituting cosine theta equals 12 over 13 and sine theta equals negative 5 over 13, this becomes the fraction with numerator 1 and denominator open paren 12 over 13 close paren squared minus open paren negative 5 over 13 close paren squared.

This can be evaluated as 169 over 119

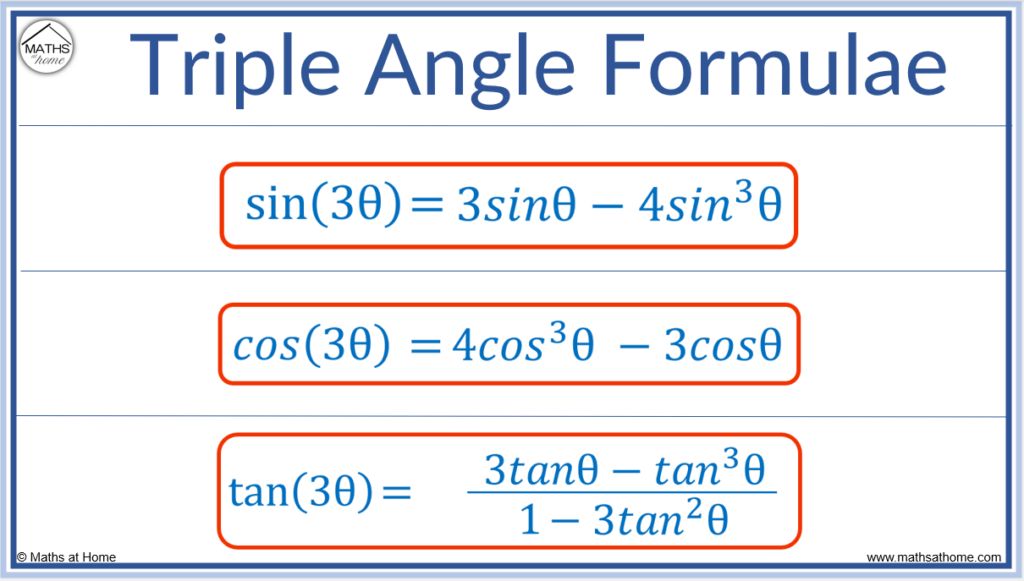
Triple Angle Formulae

The triple angle formulae are:

  • sin(3θ)=3sinθ-4sin3θ
  • cos(3θ)=4cos3θ-3cosθ
  • tan(3θ)=(3tanθ-tan3θ)/(1-3tan2θ)
triple angle formulae trigonometry

Sine Triple Angle Formula

To derive the sine triple angle formula, the addition angle formula for sine is used: the sine of open paren A plus B close paren equals sine A cosine B plus sine B cosine A.

Here, the values of A equals 2 x and B equals x are used so that the sine of open paren 2 x plus x close paren equals sine 2 x cosine x plus sine x cosine 2 x.

Then the double angle formula are used : sine 2 x equals 2 sine x cosine x and cosine 2 x equals 1 minus 2 sine squared x.

Therefore the sine of open paren 2 x plus x close paren equals sine 2 x cosine x plus sine x cosine 2 x becomes sine 3 x equals open paren 2 sine x cosine x close paren times cosine x plus the sine of x times open paren 1 minus 2 sine squared x close paren.

This simplifies to sine 3 x equals 2 sine x cosine squared x plus sine x minus 2 sine cubed x.

We can now substitute cosine squared x equals 1 minus sine squared x to obtain sine 3 x equals 2 the sine of x times open paren 1 minus sine squared x close paren plus sine x minus 2 sine cubed x.

Expanding, this becomes sine 3 x equals 2 sine x minus 2 sine cubed x plus sine x minus 2 sine cubed x.

Collecting like terms, this becomes sine 3 x equals 3 sine x minus 4 sine cubed x.

Cosine Triple Angle Formula

To derive the cosine triple angle formula, the addition formula for cosine is used: the cosine of open paren A plus B close paren equals cosine A cosine B minus sine A sine B

Here, the values of A equals 2 x and B equals x are used so that the cosine of open paren 2 x plus x close paren equals cosine 2 x cosine x minus sine 2 x sine x.

We now substitute the sine and cosine double angle formulae: sine 2 x equals 2 sine x cosine x and cosine 2 x equals cosine squared x minus sine squared x.

Therefore we obtain cosine 3 x equals open paren cosine squared x minus sine squared x close paren times cosine x minus open paren 2 sine x cosine x close paren times sine x.

Expanding, we obtain cosine 3 x equals cosine cubed x minus sine squared x cosine x minus 2 sine squared x cosine x.

We now substitute sine squared x equals 1 minus cosine squared x to obtain cosine 3 x equals cosine cubed x minus open paren 1 minus cosine squared x close paren times cosine x minus 2 times open paren 1 minus cosine squared x close paren times cosine x.

Expanding the brackets, we obtain cosine 3 x equals cosine cubed x minus cosine x plus cosine cubed x minus 2 cosine x plus 2 cosine cubed x.

Collecting like terms, we obtain cosine 3 x equals 4 cosine cubed x minus 3 cosine x.