How to Differentiate Exponential Functions

Derivatives of Exponential Functions: Video Lesson

How to Differentiate e^x

The derivative of e^x is e^x. Therefore e to the power of x remains unchanged when it is differentiated. This is the only function to have this property. The derivative of e^kx is ke^kx. For e^x, k=1 and so, the derivative of e^x is e^x.

the derivative of e to the power of x is e to the power of x

Since the derivative of e^x is just e^x, the graph of the derivative of e^x looks identical to e^x.

Exponential Function	Derivative
y=e^x	y’=e^x
y=e^kx	y’=k.e^kx
y=e^f(x)	y’=f'(x).e^f(x)
y=a^x	y’=ln(a).a^x

How to Differentiate an Exponential Function

To differentiate an exponential function, copy the exponential function and multiply it by the derivative of the power. For example, to differentiate f(x)=e^2x, take the function of e^2x and multiply it by the derivative of the power, 2x. The derivative of 2x is 2. Therefore the derivative of f(x)=e^2x is f'(x)=2e^2x .

The derivative of e^2x is 2e^2x.

The rule for differentiating an exponential function is that for f(x)=e^u, the derivative is f'(x)=u’.e^u.

u is the function found in the power of the exponential and u’ is the derivative of this function.

In words, the rule for differentiating an exponential is to multiply the original exponential function by the derivative of its power.

The rule for differentiating exponential functions is that for f(x)=e^u then f'(x)=u’.e^u, where u is the function in the power of the exponential and u’ is the derivative of this function. For f(x)=e^2x, u = 2x and u’ = 2. Therefore f'(x)=2e^2x.

Examples of Differentiating Exponential Functions

To differentiate any exponential function, differentiate the power and multiply this by the original function.

This can be written mathematically as when , .

Alternatively, this can be written as when , .

rule for the derivative of an exponential function

For example, differentiate f(x) = e^3x.

u is the power of the exponential, which is 3x.

u’ is the derivative of u. Differentiating 3x, we get u’ = 3.

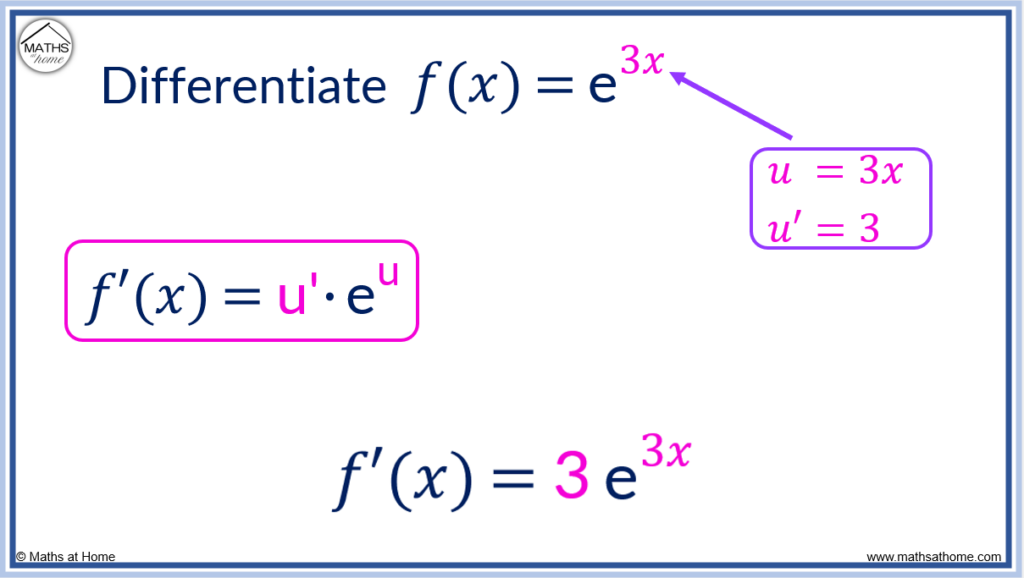
Substituting u = 3x and u’ = 3 into f'(x) = u’.e^u, we get f'(x) = 3e^3x.

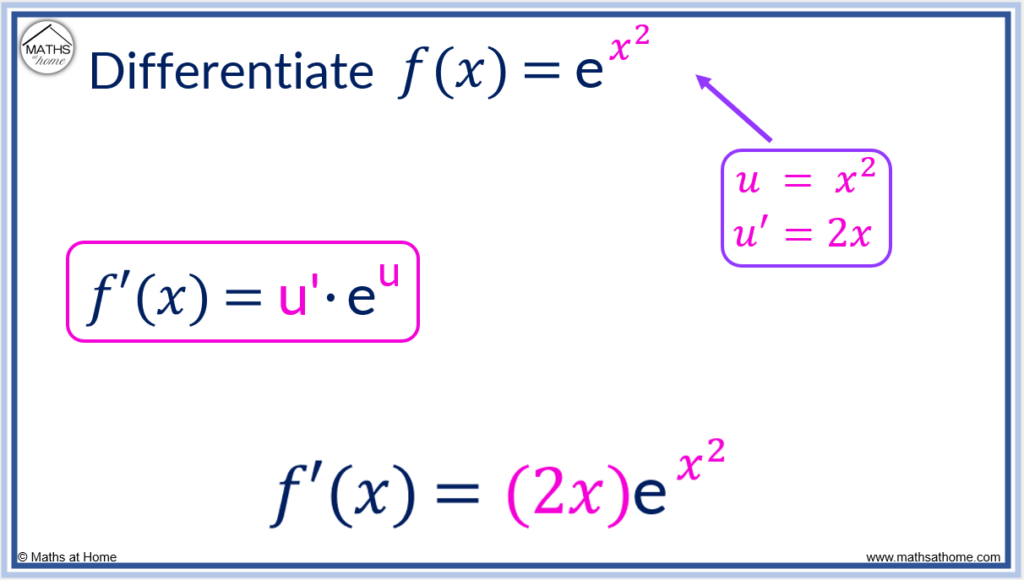
For example, differentiate f(x)=e^x².

u = x² and so, u’ = 2x.

Therefore f'(x) = u’ . e^u becomes f'(x) = (2x).e^x².

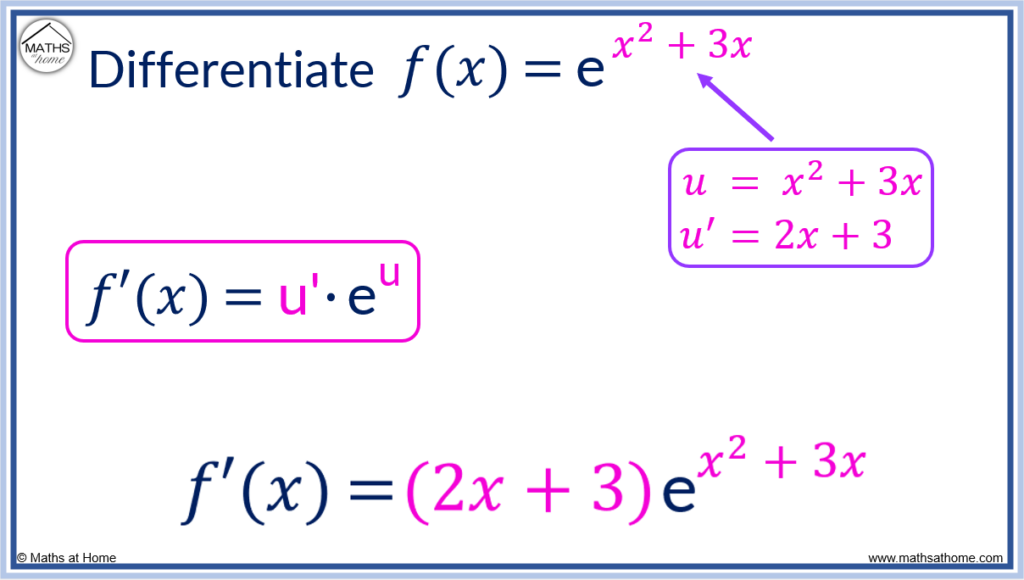
For example, differentiate f(x)=e^x²+3x .

u = x²+3x and so, u’ = 2x+3.

Therefore f'(x) = (2x+3).e^x²+3x.

how to differentiate exponential functions

For example, differentiate f(x) = e^¹/_x.

If the power of the exponential function is a fraction, rewrite it as an indice.

¹/_x can be rewritten as x^-1. Writing this fraction as an index allows us to differentiate it.

u = x^-1 and so, u’ = -x^-2.

Therefore f'(x) = (-x^-2)e^{x^-1}.

This can also be written as $f prime of x equals negative the fraction with numerator 1 and denominator x squared e raised to the 1 over x power$ or $f prime of x equals negative the fraction with numerator e raised to the 1 over x power and denominator x squared$ .

how to differentiate an exponential with a fractional power

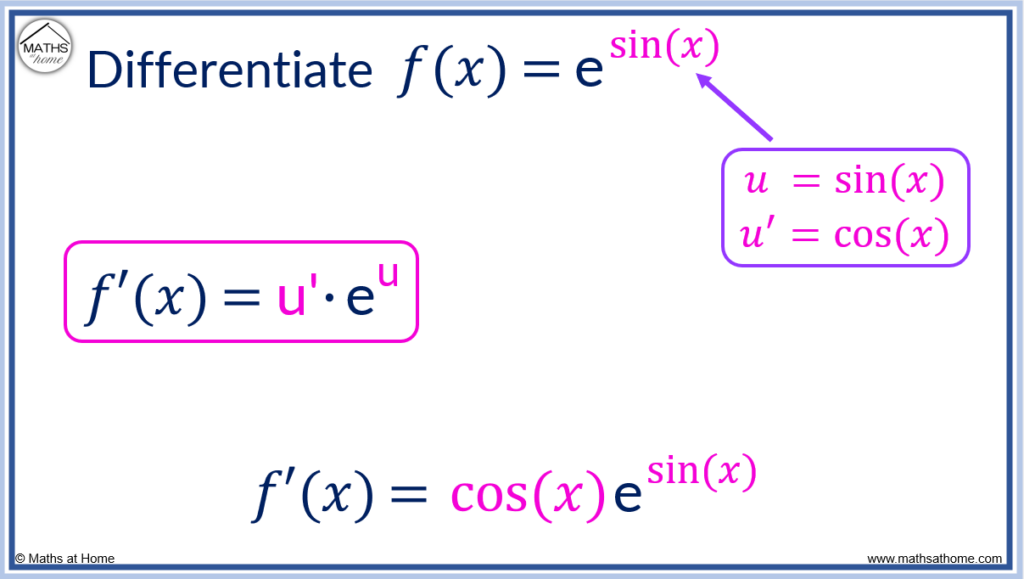
For example, differentiate e^sin(x).

u = sin(x) and so, u’ = cos(x).

Therefore f'(x) = cos(x).e^sin(x).

Here are some examples of differentiating exponential functions with solutions.

Exponential Function	Derivative
e^x	e^x
e^kx	ke^kx
e^3x	3e^3x
5e^2x	10e^2x
e^x²	(2x).e^x²
e^(2x³-x²)	(6x²-2x).e^(2x³-x²)
e^-x	-e^-x
e^sin(x)	cos(x).e^sin(x)

Chain Rule with Exponential Functions

The chain rule is used to differentiate a function of a function. The chain rule states that .

The rule for differentiating exponential functions can be used in conjunction with the chain rule.

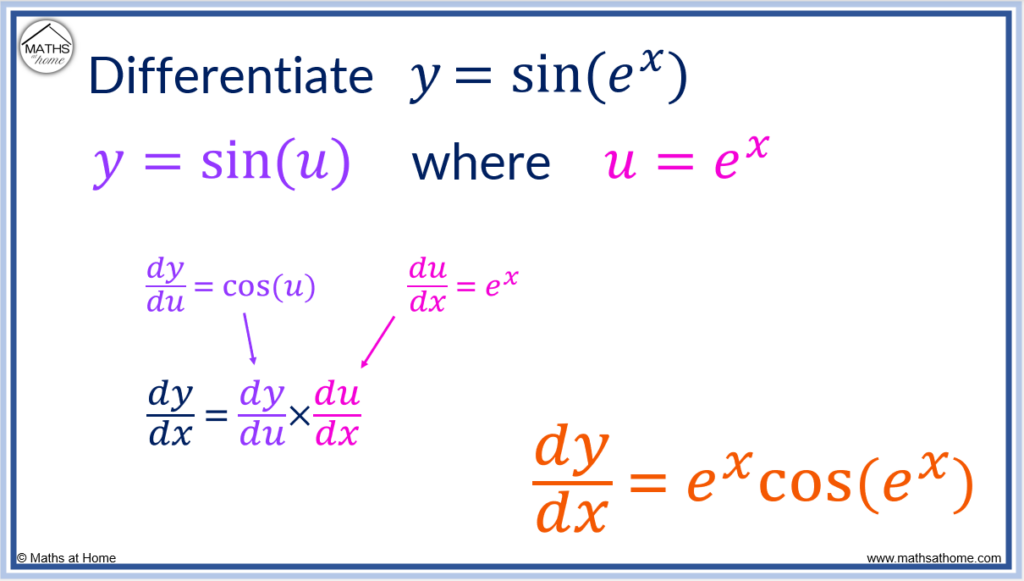
For example, differentiate y = sin(e^x).

We can write this as y = sin(u), where u = e^x.

Therefore, and .

Using the chain rule, and so, .

chain rule for differentiation involving an exponential function

Product Rule with Exponential Functions

The produce rule states that for a function , the derivative is .

Our rule for differentiating exponentials can be used alongside the product rule.

For example, differentiate y=xe^x.

Here u = x and v = e^x.

Therefore and .

Using the product rule, , the derivative is .

We can factorise out the e^x term so that .

the product rule with exponential functions

Quotient Rule with Exponential Functions

The quotient rule is $d y over d x equals the fraction with numerator v d u over d x minus u d v over d x and denominator v squared$ .

Here is an example of using the quotient rule to differentiate exponential functions.

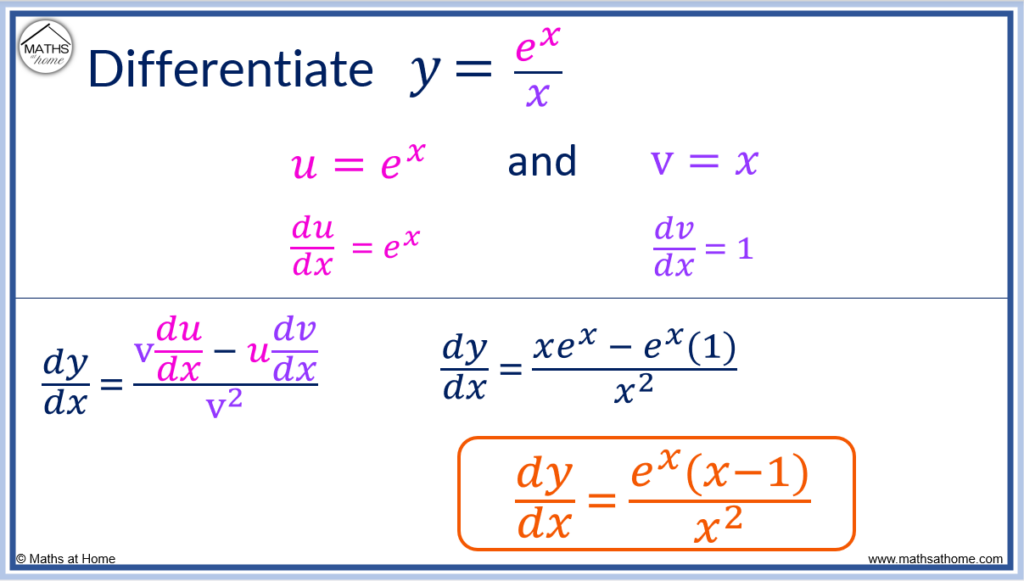
Differentiate $y equals the fraction with numerator e to the x-th power and denominator x$ .

With the quotient rule, u is the function on the numerator and v is the function on the denominator.

u = e^x and so .

v = x and so, .

Substituting these values into the quotient rule, $d y over d x equals the fraction with numerator x e to the x-th power minus e to the x-th power and denominator x squared$ .

This can be simplified by factorising out the e^x term.

$d y over d x equals the fraction with numerator e to the x-th power times open paren x minus 1 close paren and denominator x squared$ .

quotient rule to differentiate exponential functions

Implicit Differentiation of e^xy

To differentiate e^xy, use f'(x)=u’.e^u where u = xy.

Use the product rule to differentiate the power of ‘xy’ implicitly.

Implicit differentiation tells us that the derivative of y is y’.

If u = xy, the product rule gives us u’ = (1)(y)+(x)(y’) which simplifies to u’ = y + xy’.

Therefore the derivative of e^xy is (y+xy’)e^xy.

If , then $d y over d x equals the fraction with numerator y e raised to the x y power and denominator 1 minus x e raised to the x y power$ .

Use implicit differentiation to differentiate xy to get y + xy’.
Collect y’ terms together
Factorise the y’ terms
Solve the equation for y’

Proof of the Derivative of e^𝑥

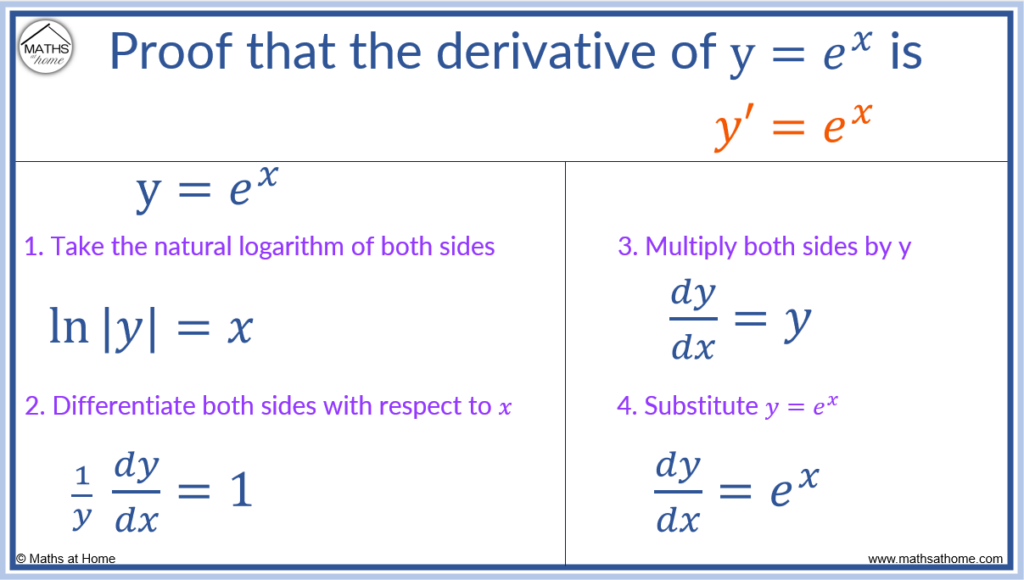
Here is an algebraic proof of why the derivative of e^x is itself.

Suppose y = e^x
Take the natural logarithm of both sides so that ln|y|=x
Differentiating both with respect to x, the result is (¹/_y)(^dy/_dx) = 1
Multiplying both sides by y, the result is ^dy/_dx = y
Substituting y = e^x, the result is ^dy/_dx = e^x

Derivative of e^𝑥 using first principles

The derivative of e^x can be found using differentiation by first principles.

The first principles formula states that the gradient function can be found using $f prime of x equals lim over h right arrow 0 the fraction with numerator f of open paren x plus h close paren minus f of x and denominator h$ .

If , then .

The term can be written as .

The first principles formula then becomes $f prime of x equals lim over h right arrow 0 the fraction with numerator e to the x-th power e raised to the h power minus e to the x-th power and denominator h$ .

The term can then be factored out to give us .

This limit can now be separated into two limits $f prime of x equals lim over h right arrow 0 of e to the x-th power times lim over h right arrow 0 of the fraction with numerator e raised to the h minus 1 power and denominator h$ .
because there are no h terms in .
Since $lim over x right arrow 0 of the fraction with numerator a. to the x-th power minus 1 and denominator x equals l n of a.$ , we can see that , $lim over h right arrow 0 of the fraction with numerator e raised to the h power minus 1 and denominator h equals l n of e$ , which equals 1.
$f prime of x equals lim over h right arrow 0 of e to the x-th power times lim over h right arrow 0 of the fraction with numerator e raised to the h minus 1 power and denominator h$ becomes

Proof of the Derivative of e^𝑥 using Series

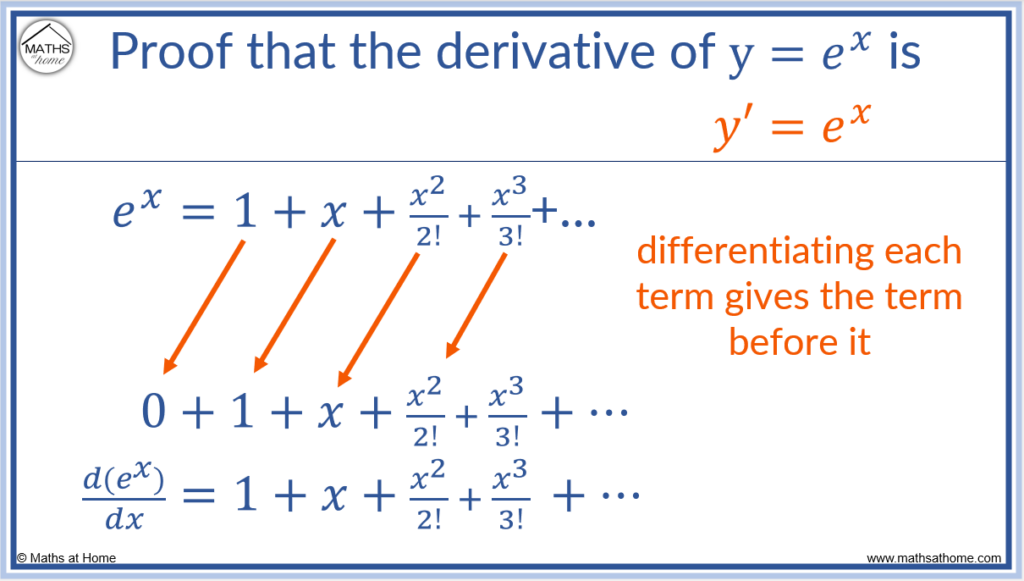
e^x can be written as a power series as $e to the x-th power equals 1 plus the fraction with numerator x and denominator 1 factorial plus the fraction with numerator x squared and denominator 2 factorial plus the fraction with numerator x cubed and denominator 3 factorial plus the fraction with numerator x to the fourth power and denominator 4 factorial plus period period period$

Each term can be differentiated to give the term before it.

For example, 1 differentiates to 0, x differentiates to 1, ^x²/₂ differentiates to x and so on.

Since there are an infinite number of terms in this power series, the series remains the same upon differentiating it.

$1 plus the fraction with numerator x and denominator 1 factorial plus the fraction with numerator x squared and denominator 2 factorial plus the fraction with numerator x cubed and denominator 3 factorial plus the fraction with numerator x to the fourth power and denominator 4 factorial plus period period period$ differentiates to $1 plus the fraction with numerator x and denominator 1 factorial plus the fraction with numerator x squared and denominator 2 factorial plus the fraction with numerator x cubed and denominator 3 factorial plus the fraction with numerator x to the fourth power and denominator 4 factorial plus period period period$